Maharashtra Board Class 11 Economics Notes Chapter 3 Partition Values

By going through these Maharashtra State Board Class 11 Economics Notes Chapter 3 Partition Values students can recall all the concepts quickly.

Maharashtra State Board Class 11 Economics Notes Chapter 1 Basic Concepts in Economics

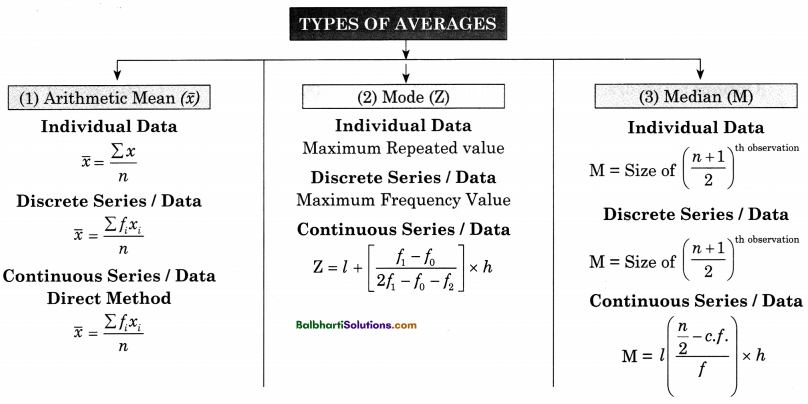
→ Types Of Averages:

(1) Arithmetic Mean ( \(\bar{x}\) ):

• Individual Data
  \(\bar{x}=\frac{\sum x}{n}\)
• Discrete Series / Data
  \(\bar{x}=\frac{\sum f_{i} x_{i}}{n}\)
• Continuous Series / Data Direct Method
  \(\bar{x}=\frac{\sum f_{i} x_{i}}{n}\)

(2) Mode (Z):

• Individual Data
  Maximum Repeated value
• Discrete Series/ Data
  Maximum Frequency Value
• Continuous Series / Data
  \(\mathrm{Z}=l+\left[\frac{f_{1}-f_{0}}{2 f_{1}-f_{0}-f_{2}}\right] \times h\)

(3) Median (M) :

• Individual Data
  M = Size of \(\left(\frac{n+1}{2}\right)^{\text {th observation }}\)
• Discrete Series I Data
  M = Size of \(\left(\frac{n+1}{2}\right)^{\text {th observation }}\)
• Continuous Series I Data
  M = \(l\left(\frac{\frac{n}{2}-c . f .}{f}\right) \times h\)

→ 29th June is celebrated as “Statistics Day” in India to recognise the contributions of noted Indian
Statistician. Prof. Prasanta Chandra Mahalanobis.

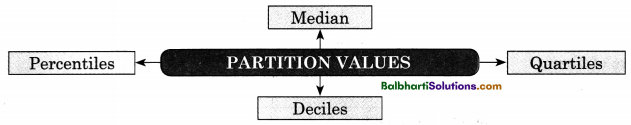
→ Partition Values : Values which divide the data into required number of equal parts are called partition value or fractiles.

→ Partition Values:

• Percentiles
• Quartiles
• Median
• Deciles

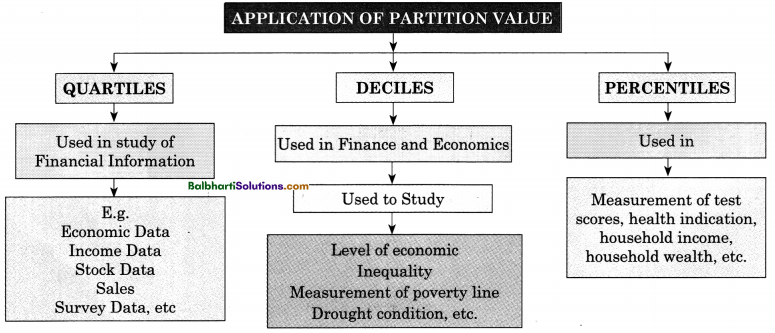
→ Application Of Partition Value:

1. Quartiles
2. Deciles
3. Percentiles

Quartiles:

• Used in study of Financial Information
• E.g.
  • Economic Data
  • Income Data
  • Stock Data
  • Sales
  • Survey Data, etc

Deciles:
Used in Finance and Economics
Used to Study:

• Level of economic
• Inequality
• Measurement of poverty line
• Drought cohdition, etc.

Percentiles:
Used in Measurement of test scores, health indication, household income, household wealth, etc.

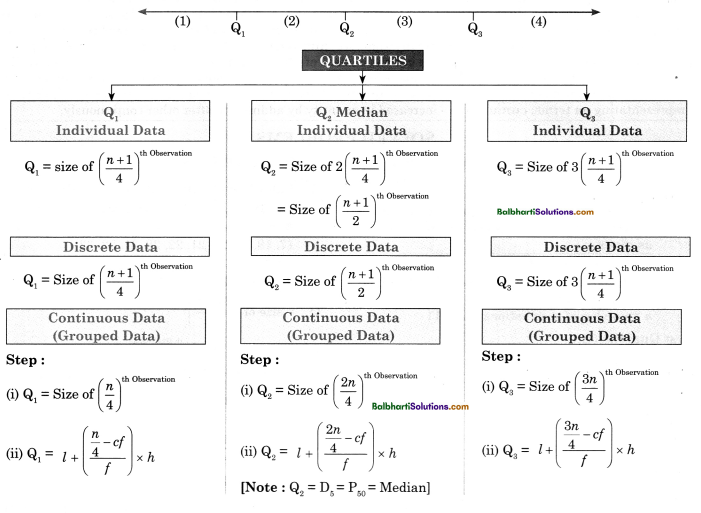
→ Quartiles : Quartiles are the values (data) which divide the series (distribution) into four equal parts. They are the 3 values that divide the distribution into 4 parts, each representing one quarters of the score. These 3 values are called as first quartile (Q₁), second quartile (Q₂) and third quartile (Q₃). Second quartile is nothing but the median.

→ Quartiles

Q₁ Individual Data:
Q₁ = size of \(\left(\frac{n+1}{4}\right)^{\text {th observation }}\)

Discrete Data:
Q₁ = size of \(\left(\frac{n+1}{4}\right)^{\text {th observation }}\)

Continuous Data (Grouped Data):

Step:

1. Q₁ = size of \(\left(\frac{n}{4}\right)^{\text {th observation }}\)
2. Q₁ = l + \(\left(\frac{\frac{n}{4}-c f}{f}\right)\) x h

Q₂ Median Individual Data:
Q₂ = size of \(\left(\frac{n+1}{4}\right)^{\text {th observation }}\)
= size of \(\left(\frac{n+1}{2}\right)^{\text {th observation }}\)

Discrete Data :
Q₂ = size of \(\left(\frac{n+1}{2}\right)^{\text {th observation }}\)

Continuous Data (Grouped Data):
Step:

1. Q₂ = size of \(\left(\frac{2n}{4}\right)^{\text {th observation }}\)
2. Q₂ = l + \(\left(\frac{\frac{2 n}{4}-c f}{f}\right)\) x h
   [Note : Q₂ = D₅ = P₅₀ Median]

Q₃ Individual Data:
Q₃ = size of 3\(\left(\frac{n+1}{4}\right)^{\text {th observation }}\)

Discrete Data:
Q₃ = size of 3\(\left(\frac{n+1}{4}\right)^{\text {th observation }}\)

Continuous Data (Grouped Data):
Step:

1. Q₃ = size of \(\left(\frac{3 n}{4}\right)^{\text {th } \text { Observation }}\)
2. Q₃ = l + \(\left(\frac{\frac{3 n}{4}-c f}{f}\right)\) x h

→ Deciles : They are the values of data which divide the whole set of observations into 10 equal parts. There are 9 points i.e. , D₁, D₂ to D₉ which divide the data into 10 equal parts. While calculating Deciles, data has to be arranged in ascending or descending order.

• Individual Data : \(\mathrm{D}_{j}=j\left(\frac{n+1}{10}\right)^{\text {th } \text { Observation }}\) [where j = 1,2, ……..9]
• Continuous Data : \(\mathrm{D}_{j}=l+\left(\frac{\frac{j n}{10}-c f}{f}\right) \times h\) [where j = 1,2, ………….9]

→ Percentiles : It divides the whole set of observations into 100 equal parts. There are 99 percentile.
They are denoted by P₁, P₂ to P₃ ………….. P₉₉ The 50th percentile is called as Median.

(i) Individual Data and Discrete Data : P_k = size of k \(\left(\frac{n+1}{100}\right)^{\text {th observation }}\) [Where k = 1, 2, ……………99]
(ii) Continuous Data : P_k = l + \(\left(\frac{\frac{k n}{100}-c f}{f}\right)\) x h [Where k = 1, 2, ……………99]

Word Meaning:

procedure – steps; arithmetic – study of numbers; mean – average; median – middle; quartiles – divided into four equal groups; deciles – divided into 10 equal groups; percentiles – divided into 100 equal groups; descriptive – to describe; poverty – poor; acquainted – to get to known; statistical – use of statistics; magnitude – in great extent; misinterprets – misunderstand; survey – observe; fluctuations – changes; inflation – increase in price; povertyline – minimum required income to get basic needs of life; drought – no rainfall in an area; portfolio investments – range of investments; bench marking – measuring the performance; baseline – minimum way tci compare; observations – the data in numbers; frequency distribution – mathematical function; symbolically – representating in terms; cumulative – increased in quantity by adding one after other continuously.