Balbharti Maharashtra State Board 11th Maths Book Solutions Pdf Chapter 6 Functions Ex 6.2 Questions and Answers.
11th Maths Part 2 Functions Exercise 6.2 Questions And Answers Maharashtra Board
Question 1.
If f(x) = 3x + 5, g(x) = 6x – 1, then find
(i) (f + g) (x)
(ii) (f – g) (2)
(iii) (fg) (3)
(iv) (f/g) (x) and its domain
Solution:
f(x) = 3x + 5, g (x) = 6x – 1
(i) (f + g) (x) = f (x) + g (x)
= 3x + 5 + 6x – 1
= 9x + 4
(ii) (f – g) (2) = f(2) – g(2)
= [3(2) + 5] – [6(2) – 1]
= 6 + 5 – 12 + 1
= 0
(iii) (fg) (3) = f (3) g(3)
= [3(3) + 5] [6(3) – 1]
= (14) (17)
= 238
(iv) \(\left(\frac{\mathrm{f}}{\mathrm{g}}\right)(x)=\frac{\mathrm{f}(x)}{\mathrm{g}(x)}=\frac{3 x+5}{6 x-1}, x \neq \frac{1}{6}\)
Domain = R – {\(\frac{1}{6}\)}
![]()
Question 2.
Let f: (2, 4, 5} → {2, 3, 6} and g: {2, 3, 6} → {2, 4} be given by f = {(2, 3), (4, 6), (5, 2)} and g = {(2, 4), (3, 4), (6, 2)}. Write down gof.
Solution:
f = {(2, 3), (4, 6), (5, 2)}
∴ f(2) = 3, f(4) = 6, f(5) = 2
g ={(2, 4), (3, 4), (6, 2)}
∴ g(2) = 4, g(3) = 4, g(6) = 2
gof: {2, 4, 5} → {2, 4}
(gof) (2) = g(f(2)) = g(3) = 4
(gof) (4) = g(f(4)) = g(6) = 2
(gof) (5) = g(f(5)) = g(2) = 4
∴ gof = {(2, 4), (4, 2), (5, 4)}
Question 3.
If f(x) = 2x2 + 3, g(x) = 5x – 2, then find
(i) fog
(ii) gof
(iii) fof
(iv) gog
Solution:
f(x) = 2x2 + 3, g(x) = 5x – 2
(i) (fog) (x) = f(g(x))
= f(5x – 2)
= 2(5x – 2)2 + 3
= 2(25x2 – 20x + 4) + 3
= 50x2 – 40x + 11
(ii) (gof) (x) = g(f(x))
= g(2x2 + 3)
= 5(2x + 3) – 2
= 10x2 + 13
(iii) (fof) (x) = f(f(x))
= f(2x2 + 3)
= 2(2x2 + 3)2 + 3
= 2(4x4 + 12x2 + 9) + 3
= 8x4 + 24x2 + 21
(iv) (gog) (x) = g(g(x))
= g(5x – 2)
= 5(5x – 2) – 2
= 25x – 12
![]()
Question 4.
Verify that f and g are inverse functions of each other, where
(i) f(x) = \(\frac{x-7}{4}\), g(x) = 4x + 7
(ii) f(x) = x3 + 4, g(x) = \(\sqrt[3]{x-4}\)
(iii) f(x) = \(\frac{x+3}{x-2}\), g(x) = \(\frac{2 x+3}{x-1}\)
Solution:
(i) f(x) = \(\frac{x-7}{4}\)
Replacing x by g(x), we get
f[g(x)] = \(\frac{g(x)-7}{4}=\frac{4 x+7-7}{4}\) = x
g(x) = 4x + 7
Replacing x by f(x), we get
g[f(x)] = 4f(x) + 7 = 4(\(\frac{x-7}{4}\)) + 7 = x
Here, f[g(x)] = x and g[f(x)] = x.
∴ f and g are inverse functions of each other.
(ii) f(x) = x3 + 4
Replacing x by g(x), we get
f[g(x)] = [g(x)]3 + 4
= \((\sqrt[3]{x-4})^{3}+4\)
= x – 4 + 4
= x
g(x) = \(\sqrt[3]{x-4}\)
Replacing x by f(x), we get
g[f(x)] = \(\sqrt[3]{f(x)-4}=\sqrt[3]{x^{3}+4-4}=\sqrt[3]{x^{3}}\) = x
Here, f[g(x)] = x and g[f(x)] = x
∴ f and g are inverse functions of each other.
(iii) f(x) = \(\frac{x+3}{x-2}\)
Replacing x by g(x), we get
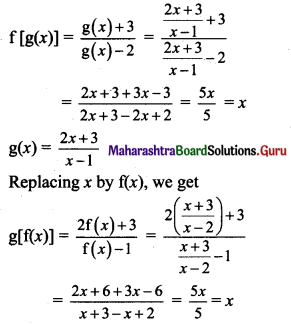
Here, f[g(x)] = x and g[f(x)] = x.
∴ f and g are inverse functions of each other.
Question 5.
Check if the following functions have an inverse function. If yes, find the inverse function.
(i) f(x) = 5x2
(ii) f(x) = 8
(iii) f(x) = \(\frac{6 x-7}{3}\)
(iv) f(x) = \(\sqrt{4 x+5}\)
(v) f(x) = 9x3 + 8
(vi) f(x) = 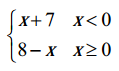
Solution:
(i) f(x) = 5x2 = y (say)
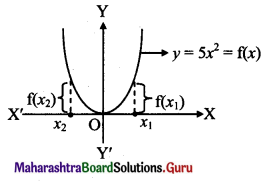
For two values (x1 and x2) of x, values of the function are equal.
∴ f is not one-one.
∴ f does not have an inverse.
(ii) f(x) = 8 = y (say)
For every value of x, the value of the function f is the same.
∴ f is not one-one i.e. (many-one) function.
∴ f does not have the inverse.
(iii) f(x) = \(\frac{6 x-7}{3}\)
Let f(x1) = f(x2)
∴ \(\frac{6 x_{1}-7}{3}=\frac{6 x_{2}-7}{3}\)
∴ x1 = x2
∴ f is a one-one function.
f(x) = \(\frac{6 x-7}{3}\) = y (say)
∴ x = \(\frac{3y+7}{6}\)
∴ For every y, we can get x
∴ f is an onto function.
∴ x = \(\frac{3y+7}{6}\) = f-1 (y)
Replacing y by x, we get
f-1 (x) = \(\frac{3x+7}{6}\)
(iv) f(x) = \(\sqrt{4 x+5}, x \geq \frac{-5}{4}\)
Let f(x1) = f(x2)
∴ \(\sqrt{4 x_{1}+5}=\sqrt{4 x_{2}+5}\)
∴ x1 = x2
∴ f is a one-one function.
f(x) = \(\sqrt{4 x+5}\) = y, (say) y ≥ 0
Squaring on both sides, we get
y2 = 4x + 5
∴ x = \(\frac{y^{2}-5}{4}\)
∴ For every y we can get x.
∴ f is an onto function.
∴ x = \(\frac{y^{2}-5}{4}\) = f-1 (y)
Replacing y by x, we get
f-1 (x) = \(\frac{x^{2}-5}{4}\)
(v) f(x) 9x3 + 8
Let f(x1) = f(x2)
∴ \(9 x_{1}^{3}+8=9 x_{2}^{3}+8\)
∴ x1 = x2
∴ f is a one-one function.
∴ f(x) = 9x3 + 8 = y, (say)
∴ x = \(\sqrt[3]{\frac{y-8}{9}}\)
∴ For every y we can get x.
∴ f is an onto function.
∴ x = \(\sqrt[3]{\frac{y-8}{9}}\) = f-1 (y)
Replacing y by x, we get
f-1 (x) = \(\sqrt[3]{\frac{x-8}{9}}\)
(vi) f(x) = x + 7, x < 0
= 8 – x, x ≥ 0
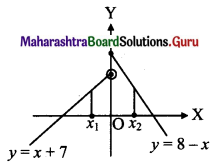
We observe from the graph that for two values of x, say x1, x2 the values of the function are equal.
i.e. f(x1) = f(x2)
∴ f is not one-one (i.e. many-one) function.
∴ f does not have inverse.
![]()
Question 6.
If f(x) = 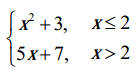 , then find
, then find
(i) f(3)
(ii) f(2)
(iii) f(0)
Solution:
f(x) = x2 + 3, x ≤ 2
= 5x + 7, x > 2
(i) f(3) = 5(3) + 7
= 15 + 7
= 22
(ii) f(2) = 22 + 3
= 4 + 3
= 7
(iii) f(0) = 02 + 3 = 3
Question 7.
If f(x) = 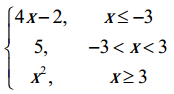 , then find
, then find
(i) f(-4)
(ii) f(-3)
(iii) f(1)
(iv) f(5)
Solution:
f(x) = 4x – 2, x ≤ -3
= 5, -3 < x < 3
= x2, x ≥ 3
(i) f(-4) = 4(-4) – 2
= -16 – 2
= -18
(ii) f(-3) = 4(-3) – 2
= -12 – 2
= -14
(iii) f(1) = 5
(iv) f(5) = 52 = 25
![]()
Question 8.
If f(x) = 2 |x| + 3x, then find
(i) f(2)
(ii) f(-5)
Solution:
f(x) = 2 |x| + 3x
(i) f(2) = 2|2| + 3(2)
= 2 (2) + 6 ….. [∵ |x| = x, x > 0]
= 10
(ii) f(-5) = 2 |-5| + 3(-5)
= 2(5) – 15 …..[∵ |x| = -x, x < 0]
= 10 – 15
= -5
Question 9.
If f(x) = 4[x] – 3, where [x] is greatest integer function of x, then find
(i) f(7.2)
(ii) f(0.5)
(iii) \(f\left(-\frac{5}{2}\right)\)
(iv) f(2π), where π = 3.14
Solution:
f(x) = 4[x] – 3
(i) f(7.2) = 4 [7.2] – 3
= 4(7) – 3 ………[∵ 7 ≤ 7.2 < 8, [7.2] = 7]
= 25
(ii) f(0.5) = 4[0.5] – 3
= 4(0) – 3 ………[∵ 0 ≤ 0.5 < 1, [0.5] = 0]
= -3
(iii) \(f\left(-\frac{5}{2}\right)\) = f(-2.5)
= 4[-2.5] – 3
= 4(-3) – 3 …….[∵-3 ≤ -2.5 ≤ -2, [-2.5] = -3]
= -15
(iv) f(2π) = 4[2π] – 3
= 4[6.28] – 3 …..[∵ π = 3.14]
= 4(6) – 3 …….[∵ 6 ≤ 6.28 < 7, [6.28] = 6]
= 21
Question 10.
If f(x) = 2{x} + 5x, where {x} is fractional part function of x, then find
(i) f(-1)
(ii) f(\(\frac{1}{4}\))
(iii) f(-1.2)
(iv) f(-6)
Solution:
f(x) = 2{x} + 5x
(i) {-1} = -1 – [-1] = -1 + 1 = 0
∴ f(-1) = 2 {-1} + 5(-1)
= 2(0) – 5
= -5
(ii) {\(\frac{1}{4}\)} = \(\frac{1}{4}\) – [latex]\frac{1}{4}[/latex] = \(\frac{1}{4}\) – 0 = \(\frac{1}{4}\)
f(\(\frac{1}{4}\)) = 2{\(\frac{1}{4}\)} + 5(\(\frac{1}{4}\))
= 2(\(\frac{1}{4}\)) + \(\frac{5}{4}\)
= \(\frac{7}{4}\)
= 1.75
(iii) {-1.2} = -1.2 – [-1.2] = -1.2 + 2 = 0.8
f(-1.2) = 2{-1.2} + 5(-1.2)
= 2(0.8) + (-6)
= -4.4
(iv) {-6} = -6 – [-6] = -6 + 6 = 0
f(-6) = 2{-6} + 5(-6)
= 2(0) – 30
= -30
![]()
Question 11.
Solve the following for x, where |x| is modulus function, [x] is the greatest integer function, {x} is a fractional part function.
(i) |x + 4| ≥ 5
(ii) |x – 4| + |x – 2| = 3
(iii) x2 + 7|x| + 12 = 0
(iv) |x| ≤ 3
(v) 2|x| = 5
(vi) [x + [x + [x]]] = 9
(vii) {x} > 4
(viii) {x} = o
(ix) {x} = 0.5
(x) 2{x} = x + [x]
Solution:
(i) |x + 4| ≥ 5
The solution of |x| ≥ a is x ≤ -a or x ≥ a
∴ |x + 4| ≥ 5 gives
∴ x + 4 ≤ -5 or x + 4 ≥ 5
∴ x ≤ -5 – 4 or x ≥ 5 – 4
∴ x ≤ -9 or x ≥ 1
∴ The solution set = (-∞, – 9] ∪ [1, ∞)
(ii) |x – 4| + |x – 2| = 3 …..(i)
Case I: x < 2
Equation (i) reduces to
4 – x + 2 – x = 3 …….[x < 2 < 4, x – 4 < 0, x – 2 < 0]
∴ 6 – 3 = 2x
∴ x = \(\frac{3}{2}\)
Case II: 2 ≤ x < 4
Equation (i) reduces to
4 – x + x – 2 = 3
∴ 2 = 3 (absurd)
There is no solution in [2, 4)
Case III: x ≥ 4
Equation (i) reduces to
x – 4 + x – 2 = 3
∴ 2x = 6 + 3 = 9
∴ x = \(\frac{9}{2}\)
∴ x = \(\frac{3}{2}\), \(\frac{9}{2}\) are solutions.
The solution set = {\(\frac{3}{2}\), \(\frac{9}{2}\)}
(iii) x2 + 7|x| + 12 = 0
∴ (|x|)2 + 7|x| + 12 = 0
∴ (|x| + 3) (|x| + 4) = 0
∴ There is no x that satisfies the equation.
The solution set = { } or Φ
(iv) |x| ≤ 3 The solution set of |x| ≤ a is -a ≤ x ≤ a
∴ The required solution is -3 ≤ x ≤ 3
∴ The solution set is [-3, 3]
(v) 2|x| = 5
∴ |x| = \(\frac{5}{2}\)
∴ x = ±\(\frac{5}{2}\)
(vi) [x + [x + [x]]] = 9
∴ [x + [x] + [x] ] = 9 …….[[x + n] = [x] + n, if n is an integer]
∴ [x + 2[x]] = 9
∴ [x] + 2[x] = 9 …..[[2[x] is an integer]]
∴ [x] = 3
∴ x ∈ [3, 4)
(vii) {x} > 4
This is a meaningless statement as 0 ≤ {x} < 1
∴ The solution set = { } or Φ
(viii) {x} = 0
∴ x is an integer
∴ The solution set is Z.
![]()
(ix) {x} = 0.5
∴ x = ….., -2.5, -1.5, -0.5, 0.5, 1.5, …..
∴ The solution set = {x : x = n + 0.5, n ∈ Z}
(x) 2{x} = x + [x]
= [x] + {x} + [x] ……[x = [x] + {x}]
∴ {x} = 2[x]
R.H.S. is an integer
∴ L.H.S. is an integer
∴ {x} = 0
∴ [x] = 0
∴ x = 0
11th Maths Practical Handbook Pdf