Class 12 English Chapter 4.3 Around the World in Eighty Days Question Answer Maharashtra Board
Balbharti Yuvakbharati English 12th Digest Chapter 4.3 Around the World in Eighty Days Notes, Textbook Exercise Important Questions and Answers.
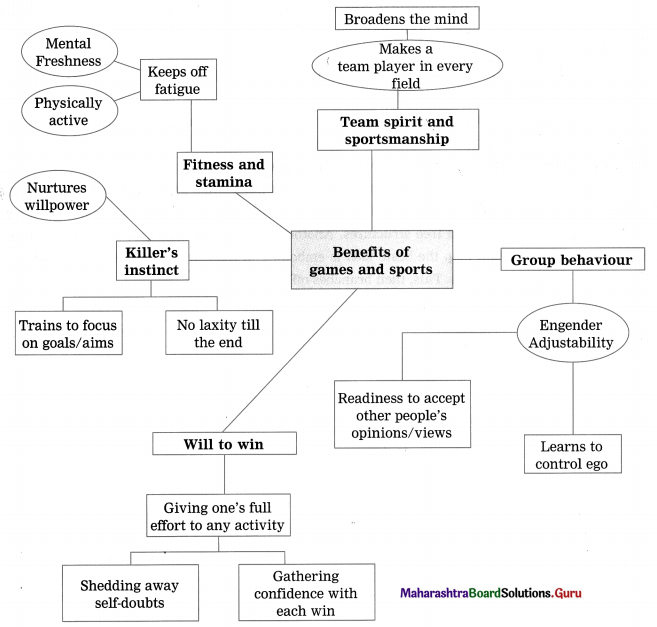
12th Std English Chapter 4.3 Brainstorming Question Answer
12th English Digest Chapter 4.3 Around the World in Eighty Days Textbook Questions and Answers
CHARACTER:
(A1)
Question (i)
One of the following is not a major character of the novel. Choose the correct one and justify.
(Select the correct one.)
(a) Phileas Fogg
(b) Aouda
(c) James Strand
(d) Jean Passepartout
Answer:
James Strand is not a major character of the novel. He was the real bank robber whom Fix was searching for.
![]()
Question (ii)
Complete the table highlighting the various traits of the major characters in the extract.
Answer:
| 1. Phileas Fogg | A solitary person; cared little about the opinions of others; honest, courageous, calm, honourable, proud; he had plenty of self-respect; was unselfish, non-materialistic, obstinate, generous. |
| 2. Aouda | Grateful, loving, concerned, sincere, sweet, noble, unselfish, non-materialistic, generous. |
| 3. Passepartout | Loyal, faithful, grateful, loving, concerned, unselfish, repentant, conscientious. |
| 4. Detective Fix | Dutiful, stupid, repentant, unimaginative, stubborn. |
Question (iii)
Phileas Fogg is as cool as a cucumber whereas Passepartout is as crazy as a loon. Explain the statement by citing some references from the extract.
Answer:
Phileas Fogg was always cool except when he hit the detective Fix. He was cool and calm in prison. Even when he felt that he had lost the wager, he shut himself up in his house quietly without any noise. He was cool when dealing with Passepartout, and also when dealing with Aouda. He could control his emotions and appear to be expressionless. Passepartout, on the other hand was a very emotional and excitable person. He was very repentant when he realized that he could have prevented Fogg’s arrest; he was thrilled when he knew that Fogg was marrying Aouda.
He was tremendously worried about Fogg when he thought that Fogg had lost the wager. Finally, when he came to know that they had reached earlier than they had thought and there was a chance that they could reach the Reform Club in time to win the bet, he became very excited. He rushed back breathlessly to inform Fogg of the fact that it was Saturday and not Sunday.
Question (iv)
Detective Fix tried hard but could not fix the charge of robbery on Fogg. Explain the statement from the point of view of Fix.
Answer:
From the point of view of Detective Fix : Scotland Yard had given only a vague description of the man who had robbed a great sum from the bank of England. I thought that Fogg fitted the description and he was the bank robber. I put obstacles in Fogg’s path just so that I could arrest him whenever I got the warrant from England. The moment we reached Liverpool, I arrested him. However, after arresting him, I found that he was not the guilty person, and the robber had already been arrested in Edinburgh some days earlier.
Fogg was a respectable gentleman living at Saville Row. There was no proof and no evidence against him except that he had been travelling all over the world apparently without any purpose. I was sorry that I arrested him wrongly and I apologized to him.
Question (v)
Describe the character sketch of Aouda from Fogg’s point of view.
Answer:
From Fogg’s point of view: Aouda has had a very difficult time in India. She was nearly killed by her persecutors, but I, Fogg, managed to save her and bring her to England with me. She is a wonderful person and so grateful for what I did. She was even apologetic for having delayed my return – she did not think about the danger she was in from her persecutors! She is very loving. She is not at all money-minded and was ready to marry me even though I did not have a penny to my name. How many will do this? What a compassionate, kind-hearted and generous person! An admirable woman, indeed, and one whom I am proud to have as my wife.
![]()
PLOT:
(A2)
Question (i)
Arrange the incidents in correct sequence as per their occurrence in the extract.
(a) Aouda accepted Fogg’s proposal of marriage.
(b) When set free, the first thing that Fogg did was he knocked Fix down.
(c) As a part of duty, Fix arrested Fogg.
(d) At the fifty-seventh second, Fogg entered the Reform Club Saloon.
Answer:
(c) As a part of duty, Fix arrested Fogg.
(b) When set free, the first thing that Fogg did was he knocked Fix down.
(d) At the fifty-seventh second, Fogg entered the Reform Club Saloon.
(a) Aouda accepted Fogg’s proposal of marriage.
Question (ii)
There is a sudden twist in the climax of the novel. Explain by citing some lines and relevant examples from the extract.
Answer:
Fogg and the reader first think that Fogg has lost not only the wager, but also the money and honour that went with it. The following lines tell us this : ‘After having steadily traversed that long journey, overcome a hundred obstacles, braved many dangers, and still found time to do some good on his way, to fail near the goal by a sudden event which he could not have foreseen, and against which he was unarmed; it was terrible!’ The unforeseen event was his arrest and imprisonment by detective Fix, who mistakenly thought he was the bank robber.
However, the twist comes when Passepartout finds out that it is not Sunday but Saturday, and perhaps they can just make it to the Reform Club in time. Just as Fogg’s antagonists are counting the seconds to their win,, Fogg manages to reach the Club and win the wager, giving a happy and thrilling ending to the novel. The following lines tell us this : ‘At the fifty-seventh second the door of the saloon opened; and the pendulum had not beat the sixtieth second when Phileas Fogg appeared, followed by an excited crowd who had forced their way through the club doors, and in his calm voice, said, “Here I am, gentlemen!”’
Question (iii)
Which of the following is an appropriate reason for Phileas Fogg starting his journey around the world?
(a) Fellow members bet Fogg
(b) Fogg bets his fellow members
(c) Fogg wants to marry Aouda
(d) Fogg committed robbery
Ans.
(a) Fellow members bet Fogg
SETTING:
(A3)
Question (i)
Choose from the following options, the means of transport used in the novel and explain the way they help the characters.
(a) Elephant
(b) Horse
(c) Train
(d) Steamer
Answer:
Elephants, trains and steamers were used as a means of transport in the novel. They help the characters go around the world in the allotted time. After overcoming a number of obstacles, they reach London in time to win the wager.
![]()
Question (ii)
The beginning of the extract is a scene in the prison at the custom house. From there the novel moves further from one place/spot to another. Pick and explain all the places/spots where the incidents took place.
Answer:
From the prison at the custom house, Fogg and his companions go to Liverpool station to catch a train back to London, where Fogg lives and where the Reform Club is located. Once they reach London, thinking that they had lost the wager, they go to Saville Row, which is Fogg’s residence. The next location is the preacher’s house where Passepartout goes to make arrangements for Fogg’s wedding. The last location is the Reform Club, which Fogg reaches in time to win his wager.
Question (iii)
Most of the setting in the extract is in London. Explain how this is suited to the theme of the novel.
Answer:
Fogg’s residence was in London. The Reform Club, which Fogg frequented on a regular basis and where the all-important wager took place, is also in London. Fogg’s journey starts from London and ends in London. Hence, London is the central place in the novel, and thus this setting is suited to the theme.
(iv) Describe the importance of the following places in the development of the plot and behaviour of the characters.
Question (a)
Liverpool.
Answer:
Liverpool is the place where Fogg disembarks from his steamer, and from where he has to catch a train to London. Liverpool is also the place where he is arrested by Fix and thus misses his train. He arranges a special train in an attempt to reach London on time.
![]()
Question (b)
London.
Answer:
Fogg lives in London. The Reform Club, which Fogg frequented on a regular basis, is also in London. Fogg’s accepts the wager in London; his journey starts from London and ends in London. Hence, London is the central place in the novel.
Question (c)
Reform Club.
Answer:
Reform Club is the place which Fogg frequented on a regular basis. It is at the Reform Club that Fogg gets involved in an argument over an article, and where the wager with his fellow club members takes place. It is the place where Fogg was supposed to return before 8.45 p.m. on 21 December, 80 days later. It is the place where his antagonists are waiting anxiously for him, and which he reaches at practically the last second to win his wager.
Question (d)
Saville Row.
Answer:
Phileas Fogg’s residence is in Saville Row.
This is also the place where he takes Aouda.
Fogg has always stayed quietly at this place.
When he returned from his trip around the world and thought he had lost the wager, he remained there so quietly that no one even knew he had returned.
Question (e)
Edinburgh.
Answer:
Detective Fix arrested Phileas Fogg in Liverpool thinking that he was a bank robber. However, the real bank robber, James Strand, had been arrested on 17th December at Edinburgh.
![]()
Question (iv)
Select the correct options:
Find from the options, the place which are not mentioned in the extract:
(a) Bombay (Now, Mumbai)
(b) Allahabad
(c) Chennai
(d) Calcutta (Now, Kolkata)
Answer:
Allahabad, Chennai
THEME:
(A4)
Question (i)
Find and explain the significance of various exciting incidents in the extract.
Answer:
The first exciting incident was when Fogg was arrested by Detective Fix and imprisoned in the Custom House. This meant that his return to London would be delayed, for he would have missed his train.
The second exciting incident was his release. This meant that he could perhaps still reach London on time.
The third exciting incident was when he tried to organize a train to London but had problems.
The fourth exciting incident was Aouda’s marriage proposal and Fogg’s acceptance of it. This showed that Aouda was not at all materialistic and extremely grateful to Fogg for what he had done. She was willing to marry Fogg even after knowing that he was penniless.
The fifth exciting incident was when Passepartout, on reaching the clergyman’s house, discovers that it is not Sunday as they had thought but Saturday, and there was a chance that they could still win the wager. The sixth most exciting incident was Fogg’s reaching the Reform Club at the last moment and winning the wager. All the twists and turns and the climax at the end keeps the reader glued to the novel right till the end.
Question (ii)
Write 4-5 sentences on the ‘Time’ theme of the extract.
Answer:
Phileas Fogg has to go around the world in eighty days if he has to win the wager. As he and his companions struggle to do this, time foils their plans in many cases. Fogg gets arrested for no fault of his and loses precious time. He is unable to catch the train from Liverpool, and the special train he arranged too gets delayed. Fogg is afraid that he has lost the bet. However, in the end, Fogg wins the bet with seconds to spare as he gained a day when crossing the International Date Line. His journey through the time zones had gained him a day. The ultimate message is that no one can control time; time will work the way it wants to work, and humans are at its mercy.
![]()
Question (iii)
Write 4-5 sentences on the ‘Morality’ theme of the extract.
Answer:
Fogg embarks on his journey to preserve his honour and prove his worth to the men at the Reform Club. He spends nearly all of his money along the way, showing that riches are not what he is truly out for. He is honourable – when he thinks he is penniless, he does not want Aouda to marry him. Aouda, by proposing to him, shows that she is not materialistic. He forgives Passepartout his mistakes. Passepartout shows his loyalty and love for his master at every step.
In the end, when he wins the bet, he divides whatever money is left between Passepartout and Detective Fix, showing that he had no grudges against him. The writer shows that with human effort and willpower enormous obstacles can be overcome. The writer also tells us in the end that Fogg had won something more important than money, by travelling around the world. He had won a charming woman, who made him the happiest of men. The moral at the end is that love and its attainment is more important than all the challenges and money in the world.
Question (iv)
Write the central idea of the given extract of the novel, ‘Around the World in Eighty Days’.
Answer:
The central idea of the given extract is how Fogg ultimately wins the bet even though there are unexpected delays and missed trains. He had unknowingly gained a day when crossing the International Date Line, and hence he was still in time to meet the deadline. The final statement is that love and its attainment is more important than all the challenges and money in the world.
Question (v)
Justice is served/done in the end. Explain.
Answer:
Phileas Fogg is an honourable and compassionate person. He wants to win his wager of going around the world in eighty S days. He struggles against time, as well as the obstacles and delays that come his way. Finally, when he reaches Liverpool and is about to go to London to win his wager, he is arrested because he is wrongly thought to be a bank robber. Throughout this ordeal, Fogg is calm and unshakeable. Even though he thinks he has lost the wager, he does not blame anyone or lose his temper.
He tells Aouda that he cannot marry her and make her live in poverty. Justice is done unexpectedly in the end when he wins the wager. He had unknowingly gained a day when crossing the International Date Line, and hence he was still in time to meet the deadline. In the end, the writer tells us that Fogg had won something more important than money, by travelling around the world. He had won a charming woman, who made him the happiest of men. Thus, justice was done to the quiet and honourable Phileas Fogg.
![]()
LANGUAGE:
(A5)
(i) Elaborate the following quotes in the light of the extract of the novel, ‘Around the World in Eighty Days’.
Question (a)
‘Quitters never win and winners never quit’.
Answer:
Even though Fogg loses time due to being wrongly arrested and imprisoned, he makes every effort to reach London on time. He arranges a special train from Liverpool. He does not quit when he feels that there is some faint chance of a win. Later, when Passepartout discovers that it is Saturday and not Sunday, and that there is still a chance of winning, Fogg puts aside everything and rushes headlong to the Reform Club. He does not give up in spite of all the obstacles, and comes out a winner, both in winning the bet and the love of a good woman.
Question (b)
‘Time is the only solution to problems’.
Answer:
The most important theme in the extract is time. Fogg’s wager is a race against time, and his adventures illustrate repeatedly that time is fickle, and either works for or against them. In many cases, time foils their plans, when the delays build up and the train to Liverpool leaves without them. In the end, though, it is time that helps wins Fogg his bet, since they gained a day when crossing the International Date Line. The ultimate message is that no one can control time; time will work the way it wants to work, and humans are at its mercy. Time is the only solution to problems.
Question (ii)
Following are some dialogues of the major characters in the extract. Find out who the speaker is, his/her tone, style, significance, etc., of the dialogue.
Answer:
| Dialogue | Speaker | Who said to Whom | Tone, Style, Significance etc. |
| 1. “Why do you not curse me? It was my fault that-” | Passepartout | Passepartout to Phileas Fogg | Repentant; thinks himself responsible for not telling Phileas Fogg about Fix earlier |
| 2. “If Phileas Fogg had come in the 7: 23 train, he would have got here by this time. We can, therefore, regard the bet as won.” | Andrew Stuart | Andrew Stuart to the other antagonists at the Reform Club | Nervous and anxious; the men with whom Fogg had the wager were calculating whether he would still meet the deadline. |
| 3. “Sir-forgive me-a most-unfortunate resemblance-robber arrested three days ago-you-are free!” | Detective Fix | Detective Fix to Phileas Fogg | Repentant and apologetic for arresting Phileas Fogg when he was innocent. |
| 4. “Will you pardon me for bringing you to England?” | Phileas Fogg | Phileas Fogg to Aouda | Proud and honourable; asking pardon from Aouda for bringing her to England on false hopes, when he has now become poor. |
| 5. “It is for me to ask that question. You were ruined, but now you are rich again.” | Aouda | Aouda to Phileas Fogg | Honourable and dignified; says this after Fogg wins the wager, and he asks her whether she still wanted to marry him. |
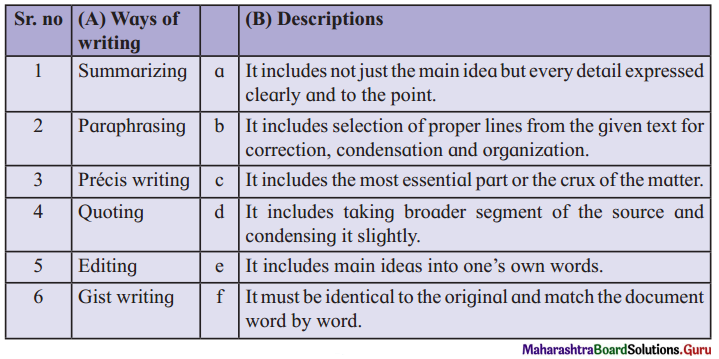
Yuvakbharati English 12th Textbook Answers Solutions Section 4 (Genre-Drama)