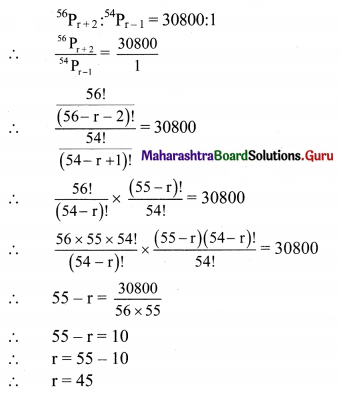
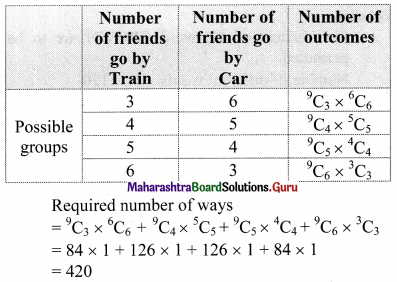
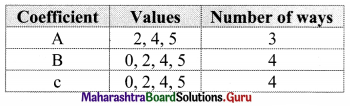
Maharashtra State Board 11th Std Secretarial Practice Textbook Solutions Digest
- Chapter 1 Secretary
- Chapter 2 Joint Stock Company
- Chapter 3 Formation of a Company
- Chapter 4 Documents Related to Formation of a Company
- Chapter 5 Members of a Company
- Chapter 6 Directors and Key Managerial Personnel of a Company
- Chapter 7 Company Meetings – I
- Chapter 8 Company Meetings – II
- Chapter 9 Business Communication Skills of a Secretary
- Chapter 10 Correspondence with Directors
- Chapter 11 Correspondence with Banks
- Chapter 12 Correspondence with Statutory Authorities