Class 11 English Chapter 1.3 The Call of the Soil Question Answer Maharashtra Board
Balbharti Yuvakbharati English 11th Digest Chapter 1.3 The Call of the Soil Notes, Textbook Exercise Important Questions and Answers.
11th Std English Chapter 1.3 Brainstorming Question Answer
11th English Digest Chapter 1.3 The Call of the Soil Textbook Questions and Answers
Question 1.
Complete the following sentences.
Answer:
- Before eating apples brought from the market, I wash and peel them off to stay away from any sort of contamination as far as possible.
- In a farmer’s market, we find rice, pulses, vegetables, fruits, dairy items, eggs, etc.
- Food adulteration means an addition of another substance to a food which may result in the loss of actual quality of food and its natural composition.
- Organic food is grown by using fertilizers made with natural ingredients, renewable resources and conservation of soil and water.
- Organic fertilizer means a type of fertilizer derived from animal matter, human and animal excreta (manure) and vegetable matter, that is, compost and crop residues.
![]()
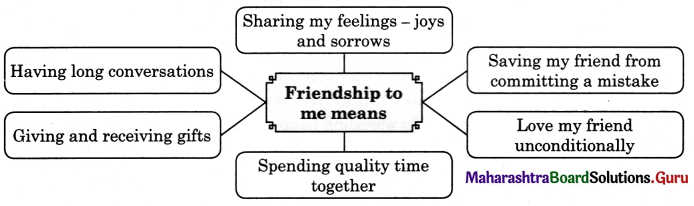
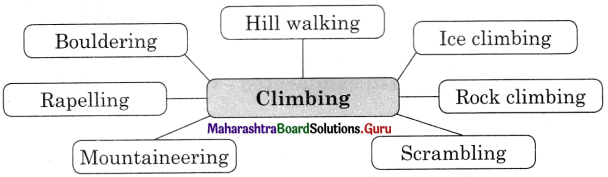
Question 2.
Complete the following web diagram.

Answer:
Question 3.
Find out from your grandparents or parents the names of vegetables and fruits they had eaten in their childhood and mention how the vegetables and fruits are different from the ones today.
Answer:
| Name of the vegetable or fruit | Shape | Colour | Taste | |
| Mango | Oblong | Yellow / Green | earlier (a) naturally ripened | now (i) artificially ripened |
| Cauliflower | Round | White with green leaves | (b) mostly available in winter and was very tasty | (ii) we get it throughout the year, but the taste is definitely worse than those available in winter |
| Apple | Round | Red / Green | (c) used to grow in natural environment and was safely given to sick people | (iii) One pays more to buy the organic varieties which are supposed to be pesticides-free and so good for health |
Question 4.
Discuss the difference between conventional farming and organic farming.
Answer:
| Conventional Farming | Organic Farming |
| (a) Relies on chemical intervention | (i) Depends on natural principles |
| (b) Farmers use synthetic and genetically modified organisms to kill pests and maximize output | (ii) No artificial ingredient or preservative is used and they are minimally processed to maintain the integrity of food |
| (c) Harms health and environment | (iii) Claimed to be healthier farm system |
![]()
(A1)
(i) Read the extract and state whether the following statements are true or false. Correct the false statements.
Question (a)
Growing in abundance is more important than the quality of the crop.
Answer:
False. The quality of the crop is more important than growing in abundance a lesser variety of crop.
Question (b)
The author wanted to grow the desi variety of rice.
Answer:
True
Question (c)
The author did not succeed in finding Kasbai.
Answer:
False. The author was successful in finding Kasbai from an Adivasi woman at Boripada, a remote village.
Question (d)
The aroma of the ‘desi’ rice would spread around the village.
Answer:
True
Question (e)
Newer hybrid crops have a great appetite for chemicals.
Answer:
True
Question (f)
The author is an example of‘reverse migration’.
Answer:
True (‘Reverse Migration’ is a new trend of shifting from bigger cities to nearby smaller towns or suburbs)
Question (ii)
Complete the flow chart.
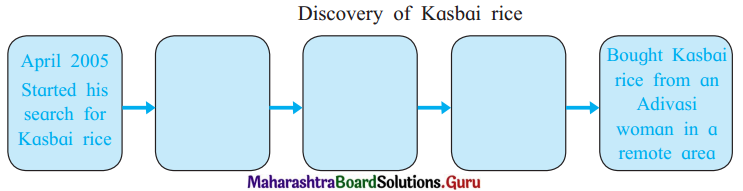
Answer:
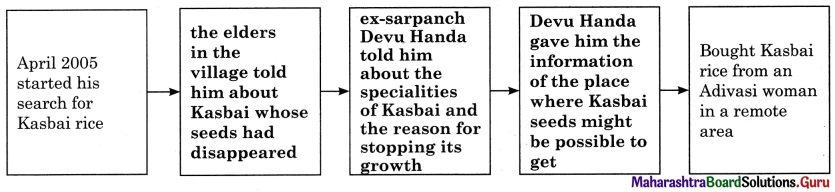
Question (iii)
Read the text and All in the blanks.
Answer:
(a) The author wanted to grow organic moong.
(b) Moru Dada wanted to spray pesticides on the moong crop.
(c) Baban’s father and some elders mentioned the name of Kasbai.
(d) “Hybrids need more water, fertilizers and pesticides”, said Devu Handa.
(e) The author bought ten kilos of rice from an Adivasi woman who lived in the remote hills.
![]()
(A2)
Question (i)
List the reactions of the agricultural officer to the author’s inquiry about Kasbai rice seeds.
Answer:
(a) He had not heard of Kasbai.
(b) He felt that the villagers were fooling the author.
(c) He mentioned the names of the latest hybrids and also offered to give him some, free of cost.
Question (ii)
Note down Devu Handa’s fond memories of Kasbai.
Answer:
Devu Handa’s fond memories of Kasbai are as follows:
- 0It needs no protection from rain
- It has an alluring aroma
- It has no hunger for chemicals like fertilizers and pesticides
(A3)
Question (i)
The writer says he grew “an awful lot of moong”. Explain the word ‘awful’ in this sentence.
Answer:
“An awful lot” is an Idiom meaning ‘a very large amount’. Everyone known to the writer did not expect a good yield of moong since he refused to spray any chemical on the plants. In spite of that, Nature did her job and the writer could get three hundred kilograms of moong which was not only ‘awful’, that means ‘large number’ but also a confidence-builder for a first-timer.
Question (ii)
The word scent is different from its synonyms aroma, fragrance or perfume. Explain how the word ‘scent’ in the subtitle ‘A Scent of Rice’, has a deeper meaning than ‘perfume’ or ‘fragrance’. Pick phrases having a similar meaning from the following:
Answer:
(i) The word ‘Scent’ in the subtitle ‘Scent of Rice’ has a deeper meaning than its usual meaning of‘fragrance’ or ‘perfume’ in the context of the text and for the author because he was trying to revive the tradition of Kasbai rice which everyone agreed had an alluring aroma. The modern farmers are forgetting conventions and have fallen a prey to hybrids. The natural ‘scent’, that is ‘flavour’ of Kasbai had drawn the writer towards real India, unspoilt by progress. The ‘scent’ was largely responsible for his quest.
(ii) Phrases
(a) In pursuit of : a quest in order to achieve something
(b) To smell a rat: to believe something wrong is happening
(c) To be keen : interested in some activity one often does and enjoys
(d) On the trail of: Trying to find someone or something by getting information about it
(e) To feel under the water : feel emotionally overwhelmed The phrases having a similar meaning of ‘A Scent of Rice’ are:
(i) In pursuit of
(ii) On the trail of
![]()
(A4)
Question 1.
She muttered in reply and we looked at Jeevan for a quick interpretation.
(A5)
Question (i)
Give reason why the text is against ‘Planting and growing more crop a year seems to be progress by normal standards.’
Answer:
Definitely planting and growing more crops a year need to be the target of the farmers but that should not be done at the cost of health hazards due to spraying of chemicals on the plants, using hybrid seeds and most importantly, forgetting our own tradition. Progress is always welcome but it should go hand in hand with safety of health and environment, importance of tradition and sentiment and last but not the least care for the rich biodiversity of our land.
Question (ii)
Describe in 150 words your experience similar to the writer’s when you pursued something and reached your goal.
Answer:
I believe “A man without an aim is like a ship without a radar”. I have been taught from my childhood to set a goal for myself. The choice of my ambition was left to me only and I was never forced to do anything.
I had multiple interests like playing football, drawing / painting and also writing about my various experiences. I started writing blogs on different social topics of my interest as well as of my own experiences in multiple walks of life. Gradually, after getting positive responses to my blogs, I got the confidence of writing short stories which were quite well accepted too by my friends and relatives. That gave me immense faith to choose writing as my profession.
One day, I saw an advertisement of ‘Crossword’ bookstore asking young writers to take part in a story-writing competition. About forty stories will be published as a book titled “ I want to be an author” without thinking twice, I took part in the competition and the rest is history. Today, I am one of those forty-one young story-tellers.
Question (iii)
The writer goes in search of an invaluable indigenous variety of seeds. List three reasons for the importance of keeping records of our indigenous agricultural practices.
Answer:
The reasons for the importance of keeping records of our indigenous agricultural practices are:
(i) (a) Reducing environmental stress by channelling water from mountain peaks to areas of demand and this practice has been going on for centuries.
(b) Using natural fertilizers made from cow dung, cow urine, evaporated cane juice of raw sugar and water to save sick plants. This practice has been mentioned in the vedas.
(ii) Traditional techniques like crop rotation, erosion prevention, systematic use of composts and manures lead to natural agricultural practices and promise better yields.
(iii) (a) Chemical fertilizers lead to degraded soil condition, deterioration in the health of quality of crops and livestock.
(b) Organic agriculture combined with tradition, innovation and science, benefits the health of the soil, people and the environment.
![]()
Question (iv)
Write a blog in about 100 to 150 words on organic farming.
Answer:
Organic Farming – A Blessing or Curse
Early 20th century saw the birth of organic farming which is an alternative to agricultural system in reaction to rapidly changing farming practices. It encourages the use of naturally occurring substances and strictly prohibits synthetic substances. Organic farming allows organically made fertilizers like compost manure, green manure and bone meal manure. Though they do not stop the use of some naturally occurring pesticides but synthetic fertilizers and pesticides are strongly prohibited. They are in favour of techniques like crop rotation, biological pest control, mixed cropping and nurturing insect killers.
Organic farming methods are internationally regulated since it has proved advantageous for health and food safety, food security, soil fertility and most importantly sustainability and biodiversity. That is why, the demand for organic farming has been steadily growing and is being encouraged all over the world. Organic farmers believe that agriculture was practised for thousands of years without the use of chemicals. If it was possible then, nothing should be impossible now.
Question (v)
Write a short paragraph in about 120 words, to be used as Counter-View for the following topic. ‘Buy a bigger cloth for your coat’.
View Section:
(a) We cannot survive by the dictum ‘Cut your cloth according to your coat’ in today’s world.
(b) In the modern world we should ‘Think Big’
(c) Think of increasing your income instead of reducing your needs.
(d) We cannot deny ourselves, what the new world offers us.
Answer:
Counter-view:
Today’s world is full of attractions. But we need to he careful about spending according to our means. We have to be prudent (wise) enough to save for the rainy days so that we don’t have to beg or borrow during emergency. It is, of course, essential to think big but at the same time over-ambition should not lead us to a point of no-return.
We should be well-aware of our capabilities and try to reach our goal judiciously instead of foolishly increasing our demands. We must make “slow but steady wins the race”, the motto of our life. Even if the new world has lots of offer, we must develop the quality of patience to wait for the right thing to come at the right time.
![]()
Question (vi)
‘Organic farming is the need of the time’. Write your views in favour and against the statement.
Answer:
| Views | Counter-views |
| 1. Reduced exposure to pesticides and chemicals since natural fertilizers are used. | (a) Some organic pesticides can actually have a worse environmental impact, contrary to the popular belief. |
| 2. One pays more for organic food but it is worth for a healthier diet. | (b) Organic products are costlier since production per unit is less than conventional farming. |
| 3. Better effect on the environment by reducing global warming. | (c) Organic farming may reduce global warming but because of more use of land, this practice is not sustainable with a growing world population. |
| 4. Organic food has better taste and more nutrition since they are given more time to develop and are not pumped with artificial things. | (d) Organic food generally gets spoilt faster since no preservatives are used to maintain their freshness and mishanding of products may deprive the market of their availability. |
Question (vii)
Appeal your classmate to say ‘No to Junk Food’. Write an appeal to prefer organic food over junk food.
Answer:
Attention! Attention! Attention!
My Dear Friends
Do you know what you’re putting in your mouth? You surely know, you are what you eat.
I’m here to advise you not to use your stomach as a trash can! Please Say ‘No’ To Junk Food.
But, what is a junk food? They’re all your favourites – yes, they are ! French fries, chicken nuggets, nachos, taco burgers, chips, chocolates, pizzas, cakes – Ah! all are mouth-watering, aren’t they? But they are also slow-poisoning us everyday causing obesity, various liver diseases, diabetes, blood pressure at a very young age — the list is long but the choice is yours!
Remember: Healthy organic foods bless us but junk foods mess us. Junk food which is rich in calories, fat, sugar and salt are yummier, I do agree with you. But are you ready to take such a big risk of spoiling your health and youth? Eat fresh, local and seasonal food because ‘health is wealth’, my dear friends!
Friends, you must eat to live and not live to eat. Let’s all have a healthy mind in a healthy body. It is my call, your call – our call!
![]()
(A6)
Projects:
Question (i)
Plant the seed of a flower or fruit of your choice in a pot or in your garden. Note its growth every day and maintain a diary recording its progress.
Question (ii)
Find out more career opportunities in the field of agriculture, organic farming, sales, storage, distribution and marketing research.
Yuvakbharati English 11th Digest Chapter 1.3 The Call of the Soil Additional Important Questions and Answers
Question 1.
Find words related to agriculture,
Answer:
The words related to agriculture are:
- foliage of moong
- pods
- crop
- land
- farming
- plant
- sow
- season
- seeds
- tractor
- plough
- leaves
- bloom
- field
- ground
- pesticide
- yield
- chemicals
- farm
- harvest
- fertile
- hybrids
- fertilizers
- farmers
- rice
Question 2.
The writer disagreed with Moru Dada because –
Answer:
The writer disagreed with Moru Dada because he suggested the use of some pesticides on the plants. The author was absolutely clear in his mind not to use any chemical which will be harmful for the soil, the plants and ultimately for human health. But for Moru Dada and his friends who were used to the conventional farming, this decision of the author was unthinkable.
![]()
Question 3.
The writer wanted to grow the traditional variety of rice because –
Answer:
1. It did not need very high variety of fertilizers
2. This variety is quite strong and is able to resist pests.
Question 4.
The write almost gave up hope of finding the desi variety of rice because –
Answer:
1. In spite of his regular visits to the villages around in search of a good desi variety, he was not successful,
2. Most of the farmers, especially the younger generation, in and around the village of Peth had switched over to hybrids and looked down upon the ‘desi’ variety, as they called the local scented variety of rice.
Question 5.
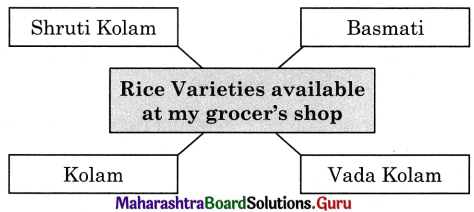
Mention the varieties of rice from the passage, “Most of the farmers…disappeared”
Answer:
- Kasbai – local long-grained scented variety
- Basmati – more aromatic than Kasbai
- Hybrids – the latest craze of the farmers.
Question 6.
List ways in which the Government officer cooperated with the author.
Answer:
1. The agricultural officer felt that the villagers were fooling the author as they had no rice by the name ‘Kasbai’ and offered to give him some latest hybrid seeds free of cost for a trial.
2. The officer-in-charge of Adivasi Mahamandal at Kasa informed the author that he remembered buying Kasbai rice a few years ago.
3. The second gentleman definitely had more knowledge of rice which he shared with the author.
Question 7.
Find synonyms of the word ‘insipid’.
Answer:
The synonyms of ‘insipid’ are:
- tasteless
- flavourless
- bland
Comprehension:
Read the extract and complete the activities given below.
Global Understanding:
Question 1.
Pick out the sentences that are true.
(i) The author thought that he would have some time to get familiar with farming.
(ii) Moru Dada was not at all sure about sowing moong in that season.
(iii) The moong seeds were brought from Gujarat.
(iv) The author did not own the land where he started farming.
Answer:
(i) True
(iii) True
![]()
Question 2.
State the advantages of the varieties as you find them in the extract.
Answer:
| Variety | Advantages |
| 1. Hybrid varieties | Short duration crop so can have two crops a year |
| 2. Traditional Rice | Good quality rice with alluring aroma |
Question 3.
Match the pairs to complete the sentences / phrases in A with the alternatives in B.
| ‘A’ | ‘B’ |
| 1. Tales of Kasbai | (a) was enlightening |
| 2. Visit to agricultural officer | (b) made us more determined to get it |
| 3. Ex-sarpanch | (c) devu Handa |
| 4. Hybrid | (d) insipid |
Answer:
| ‘A’ | ‘B’ |
| 1. Tales of Kasbai | (b) made us more determined to get it |
| 2. Visit to agricultural officer | (a) was enlightening |
| 3. x-sarpanch | (c) devu Handa |
| 4. Hybrid | (d) insipid |
Say whether the following statements are true or false. Correct the false statement.
Question 1.
The old woman weighed 10 kg of rice and gave it to the author.
Answer:
False. The old woman did not have a weighing scale.
Question 2.
The author and his friend did not know the local dialect very well.
Answer:
True
Question 3.
The people at Boripada village were well-aware of the modern way of farming.
Answer:
False. The people at Boripada never heard of hybrids, fertilizers or pesticides.
Question 4.
The old woman was unhappy to get the price of the rice.
Answer:
False. She was very happy and nodded her head with a smile of approval.
Complex Factual:
Question 1.
Supply Information from the passage,
1. I stood in the middle of lush green field of _______ and _______ .
2. Around me were rows of _______ and below a dense _____ .
Answer:
1. Moong (green gram), looked around me
2. Chikoo trees, foliage of moong
![]()
Question 2.
Mention any two changes that have occurred in farming of rice.
Answer:
1. Most of the farmers in and around the village of Peth had switched over to hybrids.
2. Kasbai which is a traditional long grained rice variety with distinct aroma being a long duration crop is not preferred by the farmers.
Question 3.
Mention any two difficulties that the author faced in tracing Kasbai.
Answer:
1. The Agricultural officer didn’t know Kasbai existed. So he couldn’t help the author in procuring it.
2. He couldn’t get the seeds as farmers resorted to hybrid varieties which gave quick yield.
Question 4.
The only people who still grew Kasbai rice were –
Answer:
The adivasis in a hamlet at the foothills of the mountains in the village Asarvari.
Question 5.
Each year they had to increase the quantity of urea and pesticides as –
Answer:
The hybrid varieties had insatiable appetite for chemicals and fertilizers.
Question 6.
How was the old lady’s world unspoilt by progress?
Answer:
The old lady, who, according to the write, represents real people of India, holding on to the biodiversity of Indian land. They have been able to do this as they have never heard of hybrids, fertilizers or pesticides.
![]()
Inference / Interpretation / Analysis
Question 1.
Explain.
“I was grateful to have taken Moru’s advice”
Answer:
The author was not sure about planting moong in that season as he wanted to get familiar with the process of farming. But Moru Dada insisted that the season was right for planting moong seeds. He was right and the author was thankful for listening to his advice since he had a good harvest of moong.
Complete the following sentence.
Question 1.
The author decided to not plant hybrids because –
Answer:
The hybrid had given him low yield the previous year.
Question 2.
The seeds of Kasbai wasn’t easily available because –
Answer:
It is a long duration crop and farmers could grow two crops in that duration.
Question 3.
A visit to agricultural officer was not useful.
Answer:
The agricultural officer had not heard of the traditional long duration varieties of rice. He was aware of only the hybrid varieties that gave quick yield. So visiting the agricultural office was not useful.
![]()
Question 4.
People didn’t grow Kasbai.
Answer:
The market didn’t recognize Kasbai anymore as a variety of rice, it had lost its popularity. If they grew it they wouldn’t be able to sell it. So people didn’t grow it.
Question 5.
Write a small paragraph on the new shift to hybrid plants.
Answer:
With advent of irrigation farmers have resorted to new hybrid varieties of rice. The long duration crops are now replaced by short duration hybrids as they can plant two crops in one year. The traditional varieties have lost its existence as it requires more duration for its harvest and also will attract cattle for its pleasant aroma unlike the hybrid crops.
Question 6.
Interpret the statement: “It was difficult to keep a straight face …”
Answer:
The author was very happy to know that the old lady had Kasbai rice with her. He was unsuccessful in his search for this particular aromatic rice for months and suddenly this revelation from the old lady was very exciting for him. But he had to control his feeling since the lady had not given him the seed till then.
Question 7.
Guess why the old lady nodded her head in approval.
Answer:
The old lady did not have any clue how much to ask for a basketful of seeds which she had given to the writer. She, even did not have a weighing scale to know how much she had given. But the author gave her hundred rupees which probably she did not hope for. She happily accepted the money which might be unexpected but was definitely welcomed by her.
![]()
Personal Response
Question 1.
Name any two reasons for destruction of foliage in India today.
Answer:
Reason 1: The trees are cut to clear the land for construction of buildings.
Reason 2: The growing industries also demand deforestation.
Question 2.
Frame any four questions that you would ask a farmer if you interview him.
Answer:
- What do you usually grow in your farm,
- What are the challenges you face while pursuing farming as your sole occupation,
- What do you do on a daily basis?
- What sprays do you use?
Question 3.
Mention any four rice varieties that you have heard of.
Answer:
There are many varieties of rice in India. The most common types rice and parboiled rice. are white rice, basmati rice, emperor’s.
Question 4.
According to you how can we develop sustainability in Agriculture.
Answer:
The sustainability can be attained by the following practices.
- Rotating crops
- Planting cover crops
- Applying integrated pest management
- Embracing diversity
![]()
Question 5.
Why do you think, the author was grateful to the old lady who was ‘unspoilt by progress’?
Answer:
I think, progress has its pros and cons. It is true that we cannot sit idle when the world is progressing by leaps and bounds. But we need to recognise the adverse (ill) effects of ‘progress’ and keep them away as much as possible. That is why, the author was delighted to see the old lady who had carefully kept the tradition alive by preserving the seeds of Kasbai rice.
Language Study
Question 1.
The author thought that he would have some time for farming.
(Rewrite using the Infinitive of the underlined gerund)
Answer:
The author thought that he would have some time to farm.
Question 2.
I was trying to figure out how we should go about it.
(Place the modal auxiliary with another showing obligation)
Answer:
I was trying to figure out how we must go about it.
![]()
Question 3.
The hybrid had given him low yield the previous year.
Answer:
The hybrid had not given him high yield the previous year.
Question 4.
Kasbai is a traditional long grained rice variety.
Answer:
Kasbai is a traditional long grained rice variety, Isn’t it?
Question 5.
The entire area would have this heady aroma. (Rewrite in past perfect tense)
Answer:
The entire area had this heady aroma.
Question 6.
His eyes turned dreamy as he drifted to the past. (Make it compound sentence)
Answer:
His eyes turned dreamy and he drifted to the past.
![]()
Question 7.
“If my field alone has Kasbai it will be a treat for the cattle.” He explained
(Rewrite in reported speech)
Answer:
He explained that if his field alone had Kasbai it would be a treat for the cattle.
Question 8.
The only people who still grew it were the adivasis. (Rewrite removing ‘who’)
Answer:
Only the adivasis still grew it.
Question 9.
Her world was unspoilt by ‘Progress’.
Answer:
Progress did not spoil her world.
Question 10.
She nodded her head in approval.
Answer:
She nodded her head as she had approved.
Vocabulary
Question 1.
Match the words in column ‘A’ with their synonyms in column ‘B’.
| Words (A) | Synonyms (B) |
| 1. Foliage | (a) Careful |
| 2. Gingerly | (b) Moist |
| 3. lush | (c) Leaves of a tree |
| 4. dump | (d) Thick |
Answer:
| Words (A) | Synonyms (B) |
| 1. Foliage | (c) Leaves of a tree |
| 2. Gingerly | (a) Careful |
| 3. lush | (d) Thick |
| 4. dump | (b) Moist |
![]()
Question 2.
Write antonyms of the words from the given extract:
- Appear
- Wise
- Short duration
- Modern
Answer:
- Disappear
- Crazy
- Long duration
- Traditional
Question 3.
From one word for:
1. mixed varieties
2. a different type of something
Answer:
1. hybrids
2. aroma
3. variety
Question 4.
From the odd words from each group of words.
- Reminisce, Remember, Think, Speak.
- Mourn, Wail, Delight, Lament.
- Appeasable, Insatiable, Satisfiable, Satiable.
- City, Hamlet, Town, Village.
Answer:
- Speak
- Delight
- Insatiable
- City
![]()
Question 5.
Match the words in column ‘A’ with their synonyms in column ‘B’.
| Column‘A’ | Column ‘B’ |
| (a) biodiversity | 1. chemical used to kill harmful insects |
| (b) hybrid | 2. plant food |
| (c) pesticide | 3. existence of wide variety of plants and animals on earth |
| (d) fertilizers | 4. mixed-breed |
Answer:
| Column‘A’ | Column ‘B’ |
| (a) biodiversity | 3. existence of wide variety of plants and animals on earth |
| (b) hybrid | 4. mixed-breed |
| (c) pesticide | 1. chemical used to kill |
| (d) fertilizers | 2. plant food |
Grammar:
Question 1.
Find out some simple, complex and compound sentences from the text.
Answer:
(i) Simple Sentences
- At that point, I could not have asked for anything more.
- Moru Dada rented his tractor to plough the land.
- We started to look for a good variety of traditional rice.
- The officer-in-charge here had more knowledge of rice.
(ii) Complex Sentences
- Kasbai is a traditional long-grained rice variety which has a distinct aroma.
- My bare feet were muddy as I walked around gingerly.
- We were clear that we would not use any chemicals.
- Even when there were flash floods in the sixties, Kasbai had stood its ground.
(iii) Compound Sentences.
- They just grew their rice and ate what they got.
- It was just before sunrise and the sky was turning a bright orange.
- I made a quick trip to Surat and bought around 10 kilograms of moong.
- Nature did her job and she needed no bribes.
Question 2.
List of subordinating conjunctions.
Answer:
All wh-words like, who, what, when, whom, where, why, how though / although unless since, as, that, because, while, whereas before, whichever, whoever, after etc., are subordinating conjunctions.
![]()
Glossary:
- lush – dense / thick
- damp – wettish / moist
- dense – thickest
- pods – shells
- exhilarated – very happy
- plough – cultivate
- thrilled – excited
- hibiscus – a type of flower
- yield – amount produced
- convincing – persuasive
- fertile – productive / rich
- morale – confidence
- booster – uplift / encouragement
- hybrids – mixed breeds
- aroma – scent / smell
- pleasantries – polite talks
- tremble – shiver / shake
- alluring – attractive / fascinating
- fall in line (phr) – agree
- rued – regretted bitterly
- hamlet – small village
- ditches – watercourses / canals /drains
- scrambling – climbing / moving with difficulty
- gravels – pebbles / stones
- wrinkled – covered with lines
- muttered – whispered/spoke in an undertone
- scowled – looked angrily
- nestling – naturally / pleasantly located house
- hustle and bustle (phr) – excited activities
- biodiversity – all the varieties of life on earth, their communities
- habitat fuzz – a soft covering
Yuvakbharati English 11th Textbook Answers Solutions Section 1 (Prose)


 Answer:
Answer: